
An infinite number of solutions can result from several situations. The same is true for dependent systems of equations in three variables. We know from working with systems of equations in two variables that a dependent system of equations has an infinite number of solutions.
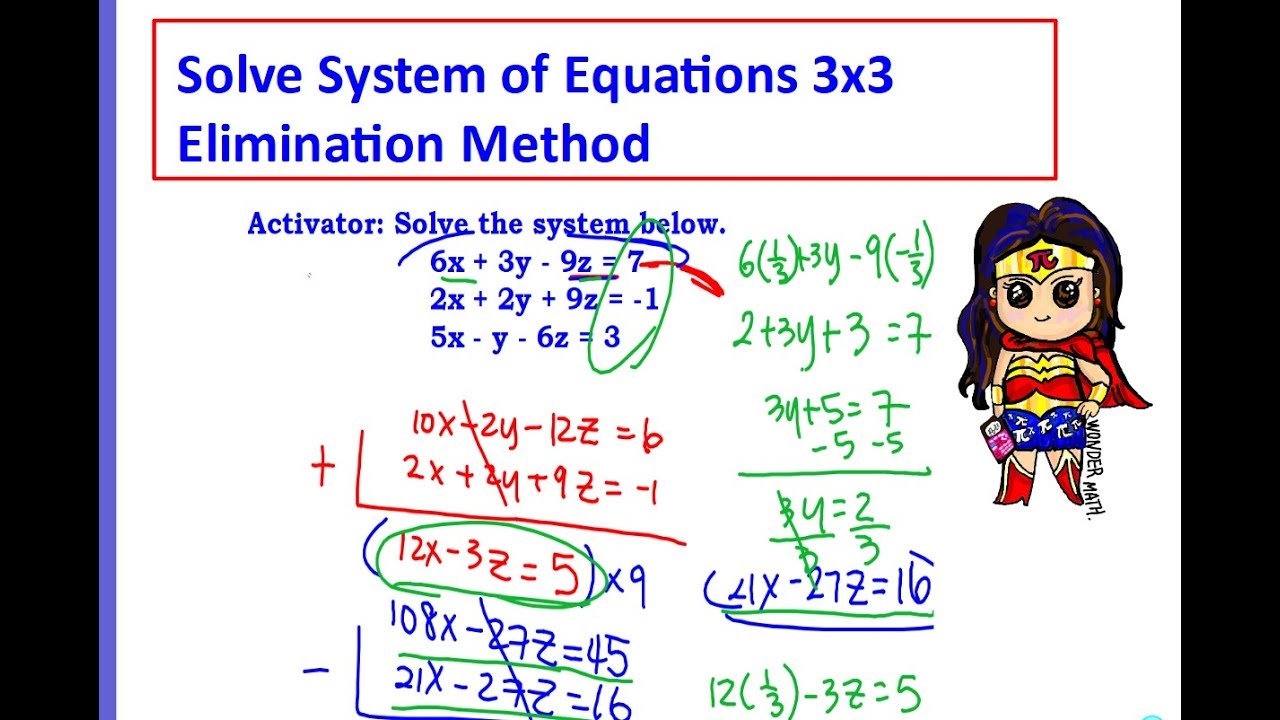
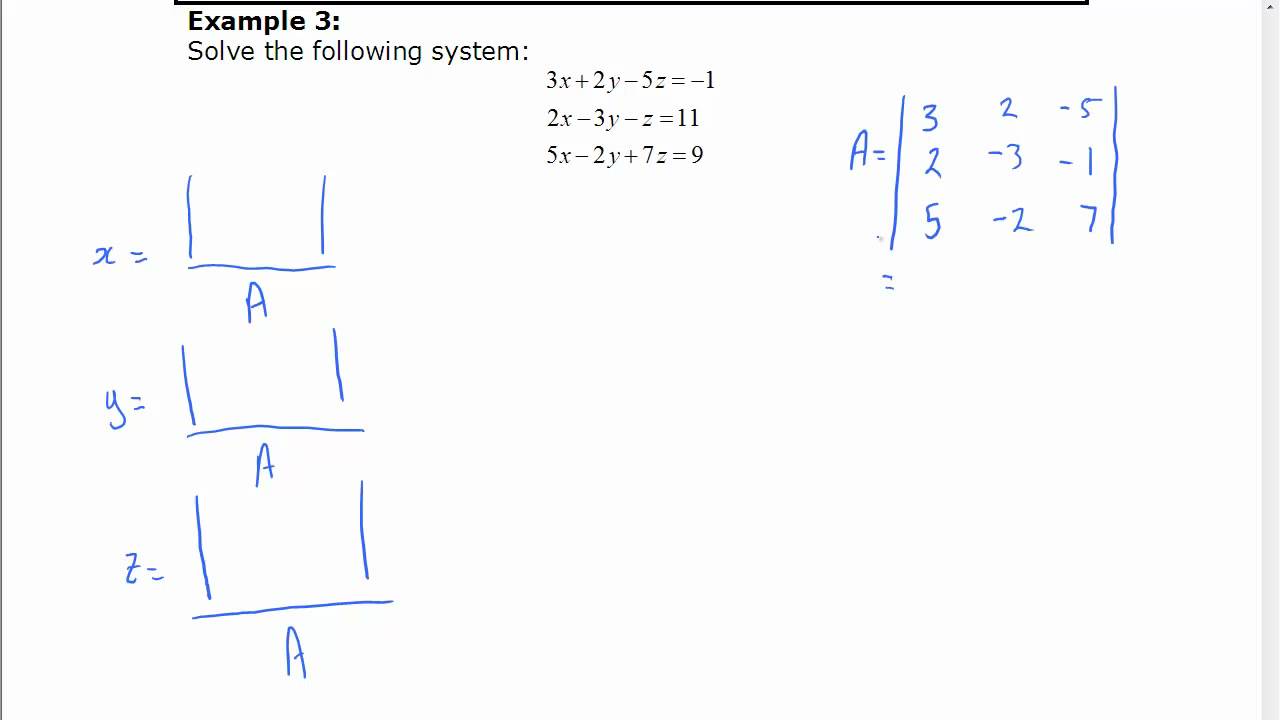
Graphically, a system with no solution is represented by three planes with no point in common.ĭependent Systems of Equations with Three Variables Inconsistent systems have no solution.Graphically, the solutions fall on a line or plane that is the intersection of three planes in space. Dependent systems have an infinite number of solutions.Graphically, the ordered triple defines a point that is the intersection of three planes in space. This is a set of linear equations, also known as a linear system of equations, in three variables: The introduction of the variable z means that the graphed functions now represent planes, rather than lines. In a system of equations in three variables, you can have one or more equations, each of which may contain one or more of the three variables, usually x, y, and z. Graphically, the solution is where the functions intersect. A solution to a system of equations is a particular specification of the values of all variables that simultaneously satisfies all of the equations. This set is often referred to as a system of equations. In mathematics, simultaneous equations are a set of equations containing multiple variables. system of equations: A set of equations with multiple variables which can be solved using a specific set of values.system of equations in three variables: A set of one or more equations, each of which may contain one ore more of the three variables usually x, y, and z.The elimination method involves adding or subtracting multiples of one equation from the other equations, eliminating variables from each of the equations until one variable is left in each equation.The graphical method involves graphing the system and finding the single point where the planes intersect.Repeat until there is a single equation left, and then using this equation, go backwards to solve the previous equations. The substitution method involves solving for one of the variables in one of the equations, and plugging that into the rest of the equations to reduce the system.In a system of equations in three variables, you can have one or more equations, each of which may contain one or more of the three variables, usually x, y, and z.


 0 kommentar(er)
0 kommentar(er)
